Foreword

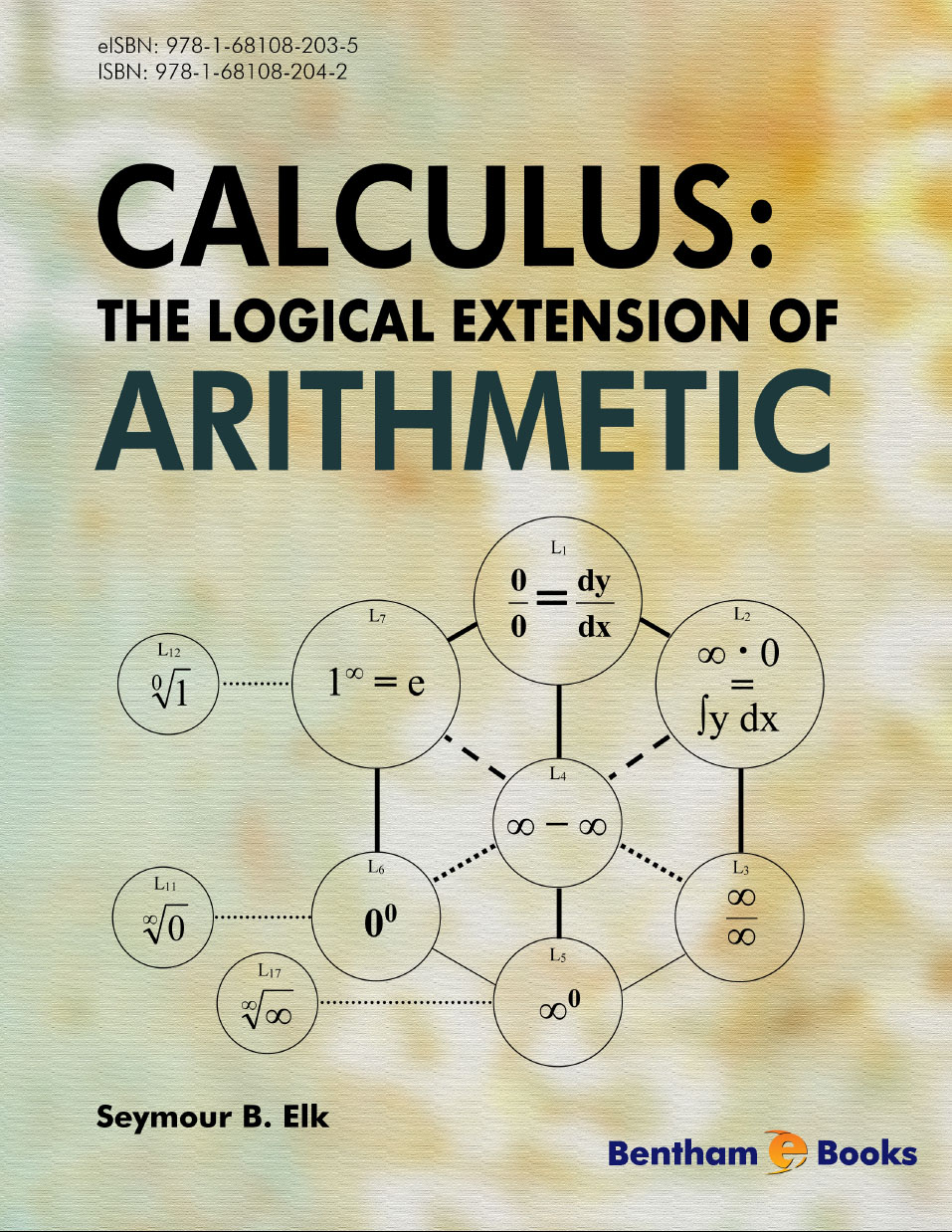
The main objective of this book is to describe a novel way of teaching elementary single-variable calculus that emphasizes an algebraically based formulation of the usual concepts of differentiation and integration. Actually, the novelty lies mainly in the emphasis and interpretation of the time-honored fundamentals of the calculus, not really in the underlying details. A central point of the pedagogical approach, which covers the usual topics treated in an introductory one-semester calculus course, is that such things as division by zero and multiplication by infinity need not be avoided – and are even useful - as long as they are properly defined.

After a lengthy introduction in which Dr. Elk outlines his pedagogical philosophy for teaching calculus, this treatise begins in Chapter 1 with a fairly traditional introduction to basic concepts such as functions, algebraic equations, sums, products, and inverses. It then deals with coordinate systems, emphasizing that the choices are far richer than merely Cartesian. Dr. Elk stresses that division by zero is acceptable if the “number” infinity is introduced and 0/0 is interpreted correctly. Also included are a description of the fundamentals of plane trigonometry, polar coordinates, a brief introduction to spherical trigonometry, which is rare for elementary calculus books, and the definition of logarithms as inverses of exponentials.

Chapter 2 is focused on differentiation, which is identified with the algebraic expression 0/0, if properly interpreted. This interpretation is just the usual (ε-δ) limit of a difference quotient. The main novelty in the treatment of limits is that l’Hospital’s rules are imposed a priori, and are used to handle what are called “l’Hospital indeterminate forms”, which include 0/0, ∞/∞, and 1^∞ . In keeping with this theme, Dr. Elk defines algebraic properties of ∞ that are more or less the same as done for the extended real numbers in more advanced analysis texts. After defining the derivative in terms of the limit quotient, the representation of derivatives in terms of ratios of differentials in Leibniz’s notation is treated algebraically and used to verify such properties as the chain rule in a manner similar to that used in the infinitesimal approach to calculus introduced by Abraham Robinson.

In Chapter 3, Dr. Elk introduces integrals interpreted as ∞⋅0 defined in a “constrained way”, which is essentially just the usual limit of Riemann sums. This is followed by a description of some of the basic methods of integration such as substitution and integration by parts. An interesting and unusual aspect of the approach is that the fundamental theorem of integral calculus is taken as an intuitive property of integration. The author covers some topics in this chapter that are rarely seen in elementary texts such as the Dirac delta distribution (“function”) as the (distributional) derivative of the Heaviside step function, and a brief intuitive description of Lebesgue integration. Chapter 4 contains a fairly traditional treatment of analytic geometry with a focus on quadratic curves and surfaces.

In Chapter 5, Dr. Elk uses the indeterminate 1^∞ as a focal point for making connections among the base of the natural logarithm, and integral representation of natural logarithms and l’Hospital’s rule. These themes are nicely interwoven and used as a source of logarithmic differentiation. The chapter ends with a very clear treatment of hyperbolic functions that stresses analogies with the trigonometric functions.

Vectors are covered in Chapter 6 in some rather unique ways. For example, there is a rather lengthy discussion on how one vector may be moved to a vector at another position in a manner that is suggestive of the concept of parallel translation. Another non-traditional and rather interesting aspect of this chapter is the way in which dyadic and triadic products of vectors are used to define vector double and triple products such as the dot, cross, vector triple and scalar triple products.

Infinite sequences and series are covered in Chapter 7. One novelty here is that the author delves into uncountable sequences, which leads to further consideration – albeit brief – of the concepts of cardinal and ordinal numbers, culminating with an intuitive description and comparison of the cardinality of the integers and the real line. The standard material on convergence tests, comparison tests and power series is covered as well.

In summary, Dr. Elk has presented a clear, lively and thought-provoking alternative to the traditional way of teaching elementary calculus, which might prove to be a viable replacement for current practice. Only time will tell if Dr. Elk’s vision resonates with mathematical educators and students.